Probability

 

Probability Definition in Math

Probability is a measure of the likelihood of an event to occur. Many events cannot be predicted with total certainty. We can predict only the chance of an event to occur i.e., how likely they are going to happen, using it. Probability can range from 0 to 1, where 0 means the event to be an impossible one and 1 indicates a certain event. Probability for Class 10 is an important topic for the students which explains all the basic concepts of this topic. The probability of all the events in a sample space adds up to 1.

For example, when we toss a coin, either we get Head OR Tail, only two possible outcomes are possible (H, T). But when two coins are tossed then there will be four possible outcomes,  i.e {(H, H), (H, T), (T, H), (T, T)}.

Download this lesson as PDF: –Download PDF Here

Formula for Probability

The probability formula is defined as the possibility of an event to happen is equal to the ratio of the number of favourable outcomes and the total number of outcomes.

Probability of event to happen P(E) = Number of favourable outcomes/Total Number of outcomes

Sometimes students get mistaken for “favourable outcome” with “desirable outcome”. This is the basic formula. But there are some more formulas for different situations or events.

Solved Examples

1) There are 6 pillows in a bed, 3 are red, 2 are yellow and 1 is blue. What is the probability of picking a yellow pillow?

Ans: The probability is equal to the number of yellow pillows in the bed divided by the total number of pillows, i.e. 2/6 = 1/3.

2) There is a container full of coloured bottles, red, blue, green and orange. Some of the bottles are picked out and displaced. Sumit did this 1000 times and got the following results:

• No. of blue bottles picked out: 300
• No. of red bottles: 200
• No. of green bottles: 450
• No. of orange bottles: 50

a) What is the probability that Sumit will pick a green bottle?

Ans: For every 1000 bottles picked out, 450 are green.

Therefore, P(green) = 450/1000 = 0.45

b) If there are 100 bottles in the container, how many of them are likely to be green?

Ans: The experiment implies that 450 out of 1000 bottles are green.

Therefore, out of 100 bottles, 45 are green.

Probability Tree

The tree diagram helps to organize and visualize the different possible outcomes. Branches and ends of the tree are two main positions. Probability of each branch is written on the branch, whereas the ends are containing the final outcome. Tree diagrams are used to figure out when to multiply and when to add. You can see below a tree diagram for the coin:

Types of Probability

There are three major types of probabilities:

• Theoretical Probability
• Experimental Probability
• Axiomatic Probability

Theoretical Probability

It is based on the possible chances of something to happen. The theoretical probability is mainly based on the reasoning behind probability. For example, if a coin is tossed, the theoretical probability of getting a head will be ½.

Experimental Probability

It is based on the basis of the observations of an experiment. The experimental probability can be calculated based on the number of possible outcomes by the total number of trials. For example, if a coin is tossed 10 times and head is recorded 6 times then, the experimental probability for heads is 6/10 or, 3/5.

Axiomatic Probability

In axiomatic probability, a set of rules or axioms are set which applies to all types. These axioms are set by Kolmogorov and are known as Kolmogorov’s three axioms. With the axiomatic approach to probability, the chances of occurrence or non-occurrence of the events can be quantified. The axiomatic probability lesson covers this concept in detail with Kolmogorov’s three rules (axioms) along with various examples.

Conditional Probability is the likelihood of an event or outcome occurring based on the occurrence of a previous event or outcome.

Probability of an Event

Assume an event E can occur in r ways out of a sum of n probable or possible equally likely ways. Then the probability of happening of the event or its success is expressed as;

P(E) = r/n

The probability that the event will not occur or known as its failure is expressed as:

P(E’) = (n-r)/n = 1-(r/n)

E’ represents that the event will not occur.

Therefore, now we can say;

P(E) + P(E’) = 1

This means that the total of all the probabilities in any random test or experiment is equal to 1.

What are Equally Likely Events?

When the events have the same theoretical probability of happening, then they are called equally likely events. The results of a sample space are called equally likely if all of them have the same probability of occurring. For example, if you throw a die, then the probability of getting 1 is 1/6. Similarly, the probability of getting all the numbers from 2,3,4,5 and 6, one at a time is 1/6. Hence, the following are some examples of equally likely events when throwing a die:

• Getting 3 and 5 on throwing a die
• Getting an even number and an odd number on a die
• Getting 1, 2 or 3 on rolling a die

are equally likely events, since the probabilities of each event are equal.

Complementary Events

The possibility that there will be only two outcomes which states that an event will occur or not. Like a person will come or not come to your house, getting a job or not getting a job, etc. are examples of complementary events. Basically, the complement of an event occurring in the exact opposite that the probability of it is not occurring. Some more examples are:

• It will rain or not rain today
• The student will pass the exam or not pass.
• You win the lottery or you don’t.

Also, read: 

• Independent Events
• Mutually Exclusive Events

Probability Theory

Probability theory had its root in the 16th century when J. Cardan, an Italian mathematician and physician, addressed the first work on the topic, The Book on Games of Chance. After its inception, the knowledge of probability has brought to the attention of great mathematicians. Thus, Probability theory is the branch of mathematics that deals with the possibility of the happening of events. Although there are many distinct probability interpretations, probability theory interprets the concept precisely by expressing it through a set of axioms or hypotheses. These hypotheses help form the probability in terms of a possibility space, which allows a measure holding values between 0 and 1. This is known as the probability measure, to a set of possible outcomes of the sample space.

Probability Density Function

The Probability Density Function (PDF) is the probability function which is represented for the density of a continuous random variable lying between a certain range of values. Probability Density Function explains the normal distribution and how mean and deviation exists. The standard normal distribution is used to create a database or statistics, which are often used in science to represent the real-valued variables, whose distribution is not known.

Important Topics in Maths : Probability And Statistics Probability Formulas Random Variable Probability Class 9 Probability Class 10 Probability Class 11 Probability Class 12 Important Questions Class 9 Maths Chapter 15 Probability Important Questions Class 11 Maths Chapter 16 Probability Important Questions Class 12 Maths Chapter 13 Probability

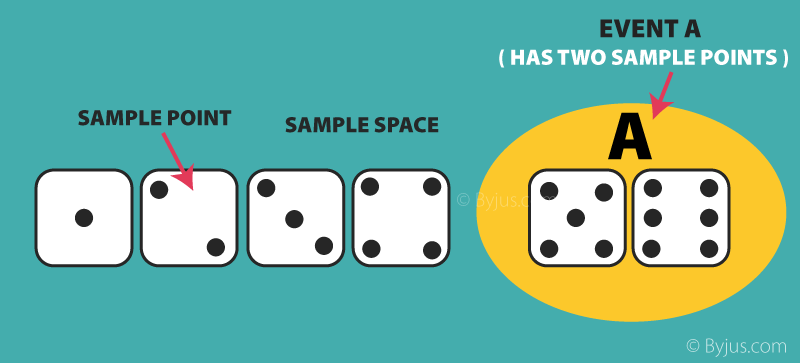
Probability Terms and Definition

Some of the important probability terms are discussed here:

Term	Definition	Example
Sample Space	The set of all the possible outcomes to occur in any trial	Tossing a coin, Sample Space (S) = {H,T} Rolling a die, Sample Space (S) = {1,2,3,4,5,6}
Sample Point	It is one of the possible results	In a deck of Cards: 4 of hearts is a sample point. The queen of clubs is a sample point.
Experiment or Trial	A series of actions where the outcomes are always uncertain.	The tossing of a coin, Selecting a card from a deck of cards, throwing a dice.
Event	It is a single outcome of an experiment.	Getting a Heads while tossing a coin is an event.
Outcome	Possible result of a trial/experiment	T (tail) is a possible outcome when a coin is tossed.
Complimentary event	The non-happening events. The complement of an event A is the event, not A (or A’)	In a standard 52-card deck, A = Draw a heart, then A’ = Don’t draw a heart
Impossible Event	The event cannot happen	In tossing a coin, impossible to get both head and tail at the same time

Applications of Probability

Probability has a wide variety of applications in real life. Some of the common applications which we see in our everyday life while checking the results of the following events:

• Choosing a card from the deck of cards
• Flipping a coin
• Throwing a dice in the air
• Pulling a red ball out of a bucket of red and white balls
• Winning a lucky draw

Other Major Applications of Probability

• It is used for risk assessment and modelling in various industries
• Weather forecasting or prediction of weather changes
• Probability of a team winning in a sport based on players and strength of team
• In the share market, chances of getting the hike of share prices

Problems and Solutions on Probability

Question 1: Find the probability of ‘getting 3 on rolling a die’.

Solution:

Sample Space = S = {1, 2, 3, 4, 5, 6}

Total number of outcomes = n(S) = 6

Let A be the event of getting 3.

Number of favourable outcomes = n(A) = 1

i.e. A  = {3}

Probability, P(A) = n(A)/n(S) = 1/6

Hence, P(getting 3 on rolling a die) = 1/6

Question 2: Draw a random card from a pack of cards. What is the probability that the card drawn is a face card?

Solution:

A standard deck has 52 cards.

Total number of outcomes = n(S) = 52

Let E be the event of drawing a face card.

Number of favourable events = n(E) = 4 x 3 = 12 (considered Jack, Queen and King only)

Probability, P = Number of Favourable Outcomes/Total Number of Outcomes

P(E) = n(E)/n(S)

= 12/52

= 3/13

P(the card drawn is a face card) = 3/13

Question 3: A vessel contains 4 blue balls, 5 red balls and 11 white balls. If three balls are drawn from the vessel at random, what is the probability that the first ball is red, the second ball is blue, and the third ball is white?

Solution:

Given,

The probability to get the first ball is red or the first event is 5/20.

Since we have drawn a ball for the first event to occur, then the number of possibilities left for the second event to occur is 20 – 1 = 19.

Hence, the probability of getting the second ball as blue or the second event is 4/19.

Again with the first and second event occurring, the number of possibilities left for the third event to occur is 19 – 1 = 18.

And the probability of the third ball is white or the third event is 11/18.

Therefore, the probability is 5/20 x 4/19 x 11/18 = 44/1368 = 0.032.

Or we can express it as: P = 3.2%.

Question 4: Two dice are rolled, find the probability that the sum is:

1. equal to 1
2. equal to 4
3. less than 13

Solution:

To find the probability that the sum is equal to 1 we have to first determine the sample space S of two dice as shown below.

S = { (1,1),(1,2),(1,3),(1,4),(1,5),(1,6)

(2,1),(2,2),(2,3),(2,4),(2,5),(2,6)

(3,1),(3,2),(3,3),(3,4),(3,5),(3,6)

(4,1),(4,2),(4,3),(4,4),(4,5),(4,6)

(5,1),(5,2),(5,3),(5,4),(5,5),(5,6)

(6,1),(6,2),(6,3),(6,4),(6,5),(6,6) }

So, n(S) = 36

1) Let E be the event “sum equal to 1”. Since, there are no outcomes which where a sum is equal to 1, hence,

P(E) = n(E) / n(S) = 0 / 36 = 0

2) Let A be the event of getting the sum of numbers on dice equal to 4.

Three possible outcomes give a sum equal to 4 they are:

A = {(1,3),(2,2),(3,1)}

n(A) = 3

Hence, P(A) = n(A) / n(S) = 3 / 36 = 1 / 12

3) Let B be the event of getting the sum of numbers on dice is less than 13.

From the sample space, we can see all possible outcomes for the event B, which gives a sum less than B. Like:

(1,1) or (1,6) or (2,6) or (6,6).

So you can see the limit of an event to occur is when both dies have number 6, i.e. (6,6).

Thus, n(B) = 36

Hence,

P(B) = n(B) / n(S) = 36 / 36 = 1

Video Lectures

Introduction

69,630

Solving Probability Questions

97,581

Probability Important Topics

1,697

Probability Important Questions

8,612

Probability Problems

1. Two dice are thrown together. Find the probability that the product of the numbers on the top of the dice is:
   (i) 6 (ii) 12 (iii) 7
2. A bag contains 10 red, 5 blue and 7 green balls. A ball is drawn at random. Find the probability of this ball being a
   (i) red ball (ii) green ball (iii) not a blue ball
3. All the jacks, queens and kings are removed from a deck of 52 playing cards. The remaining cards are well shuffled and then one card is drawn at random. Giving ace a value 1 similar value for other cards, find the probability that the card has a value
   (i) 7 (ii) greater than 7 (iii) less than 7
4. A die has its six faces marked 0, 1, 1, 1, 6, 6. Two such dice are thrown together and the total score is recorded.
   (i) How many different scores are possible?
   (ii) What is the probability of getting a total of 7?

Frequently Asked Questions (FAQs) on Probability

Q1

What is probability? Give an example

Probability is a branch of mathematics that deals with the occurrence of a random event. For example, when a coin is tossed in the air, the possible outcomes are Head and Tail.

Q2

What is the formula of probability?

The probability formula is defined as the possibility of an event to happen is equal to the ratio of the number of favourable outcomes to the total number of outcomes.

Q3

What are the different types of probability?

There are three major types of probabilities:
Theoretical Probability
Experimental Probability
Axiomatic Probability

Q4

What are the basic rules of probability?

If A and B are two events, then;
P ( A ∪ B ) = P ( A ) + P ( B ) − P ( A ∩ B )
P ( A ∩ B ) = P ( B ) ⋅ P ( A | B )

Q5

What is the complement rule in probability?

In probability, the complement rule states that “the sum of probabilities of an event and its complement should be equal to 1”. If A is an event, then the complement rule is given as:
P(A) + P(A’) = 1.

Q6

What are the different ways to present the probability value?

The three ways to present the probability values are:

• Percentage
• Ratio
• Decimal or fraction

Q7

What does the probability of 0 represent?

The probability of 0 represents that the event will not happen or that it is an impossible event.

Q8

What is the sample space for tossing two coins?

The sample space for tossing two coins is:
S = {HH, HT, TH, TT}